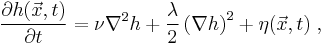Kardar–Parisi–Zhang equation
The KPZ-equation (named after its creators Mehran Kardar, Giorgio Parisi, and Vi-Cheng Zhang) is a non-linear stochastic partial differential equation. It describes the temporal change of the height  at place
at place 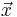 and time
and time  . It is given by
. It is given by
where 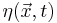 is white Gaussian noise with average
is white Gaussian noise with average  and second moment
and second moment 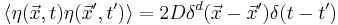 .
.  ,
,  , and
, and 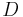 are parameters of the model and
are parameters of the model and 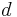 is the dimension.
is the dimension.
By use of renormalization group techniques it can be shown that the KPZ-equation is the field theory of many surface growth models, such as the Eden model, ballistic deposition, and the SOS model.
Sources
- Mehran Kardar, Giorgio Parisi, and Yi-Cheng Zhang, Dynamic Scaling of Growing Interfaces, Physical Review Letters, Vol. 56, 889 - 892 (1986). APS
- A.-L.Barabási and H.E.Stanley, Fractal concepts in surface growth (Cambridge University Press, 1995)